Measuring the Total Volume and Density of a Meteorite
Long ago, the famous mathematician Archimedes (287-212 BC) determined how to measure the volume of an object. He showed that the volume of a rock, for example, could be measured easily by immersing it in water in a calibrated beaker. The level of the water will rise because the rock displaces a volume of water equal to the rock's volume. We have all noticed this when getting into a bathtub-the level of the water rises. If you measured the amount that the water level rose and then got out and weighed yourself, you could calculate your density. (This could be disturbing, however, because the lower your density the more fat on your body!) However, placing a meteorite in a bucket of water would contaminate it by introducing trace elements, would remove soluble elements from the sample, and alter some of the minerals present. No curator would allocate samples that were going to be so mistreated.
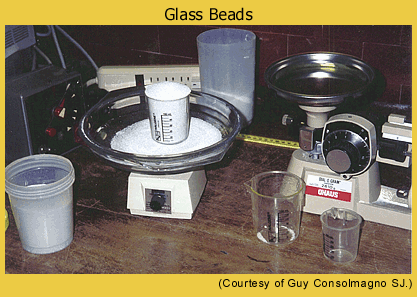
To get around the contamination and alteration problem, Consolmagno and Britt used tiny glass beads as the fluid. The beads are only 40 micrometers in diameter. They placed a sample in a beaker, poured in glass beads to overflowing, and shook the beaker on a vibrating platform to ensure that the beads filled up as much space as possible, and leveled off the pile of beads at the top of the container. They then repeated the whole procedure without the meteorite sample. They weighed the meteorite sample, the beaker with the sample and glass beads, the beaker with the glass beads only, and the beaker alone. This gives them enough information to determine what fraction of the volume of the beaker (which is also known) is occupied by the meteorite specimen, and so to calculate the volume of the sample. The density of the meteorite is equal to the meteorite mass divided by its volume.

Measuring the Volume and Density of the Grains in a Meteorite
The total (also called bulk) volume and density measured above includes the pore spaces inside each meteorite. It is also quite useful to know the "grain density" of the sample, which is equivalent to the density of the rock if absolutely no pore spaces were present. For this measurement, Consolmagno and Britt used a helium pycnometer. Pycnometers (from 'pycno,' meaning dense, and 'meter' meaning measure) are containers with precisely known volumes used to measure the density of rocks or liquids. (They borrowed theirs from the Department of Hydrology and Water Resources at the University of Arizona. Densities, and the porosities that can be derived from them, are very important parameters in understanding water flow through the surface of the Earth.)
The pycnometer consisted of two chambers, connected by a tube with a valve in it. Each chamber has assorted sensors to measure temperature and pressure, which are read out to a computer. The idea is to measure the pressure difference between the two containers, one of which has the meteorite in it.
 |
The cylindrical sample chamber in this pycnometer accommodates meteorites up to 11.5 cm (base diameter) by 24.9 cm (length). Billiard balls were used as samples of known volume with no pore spaces to assess the uncertainties in the measurements. |
Consolmagno and Britt made measurements using the following procedure:
First, they placed a meteorite sample in the sample chamber. Both chambers were then flushed with helium at one atmosphere pressure, so that no air was in either chamber. Helium, because it is a very small atom, penetrates all the cracks and holes in the rock, and all the boundaries between mineral grains, but does not get inside the grains.
Second, they closed the valve between the two chambers, and pressurized the reservoir chamber with helium to about double the atmospheric pressure.
Third,the computer recorded the temperature and pressure of each chamber every few seconds over a period of a several minutes, resulting in very precise measurements.
Fourth,the valve between the two chambers was opened and the helium in the reservoir, which was at a higher pressure than in the sample chamber, expanded into the sample chamber. The temperature and pressure were measured again. The new pressure compared to the original pressure is a measure of the ratio of the volume of open space in each chamber.
Fifth, Consolmagno and Britt repeated the entire procedure about five times for each sample.
The volumes of the two chambers are known very precisely, and the difference in volumes available to the gas is due to the presence of the sample in one of the chambers. The difference in the volume of open space between the two chambers is related to the differences in the pressures. So, Consolmagno and Britt were able to calculate the volume of grains in the sample from the pressures and the volumes of the two chambers. The density of the grains (called the grain density) is calculated from the bulk volume of the sample divided by the volume of grains, times the bulk density. The porosity of the sample is the percentage difference between the grain volume and the bulk volume, divided by the bulk volume.
Imagine repeating this elaborate procedure a couple of hundred times!